In quadratic equations, we commonly find the x-intercepts. These values tell us where the graph cuts the x-axis. The x-intercepts can also be called roots.
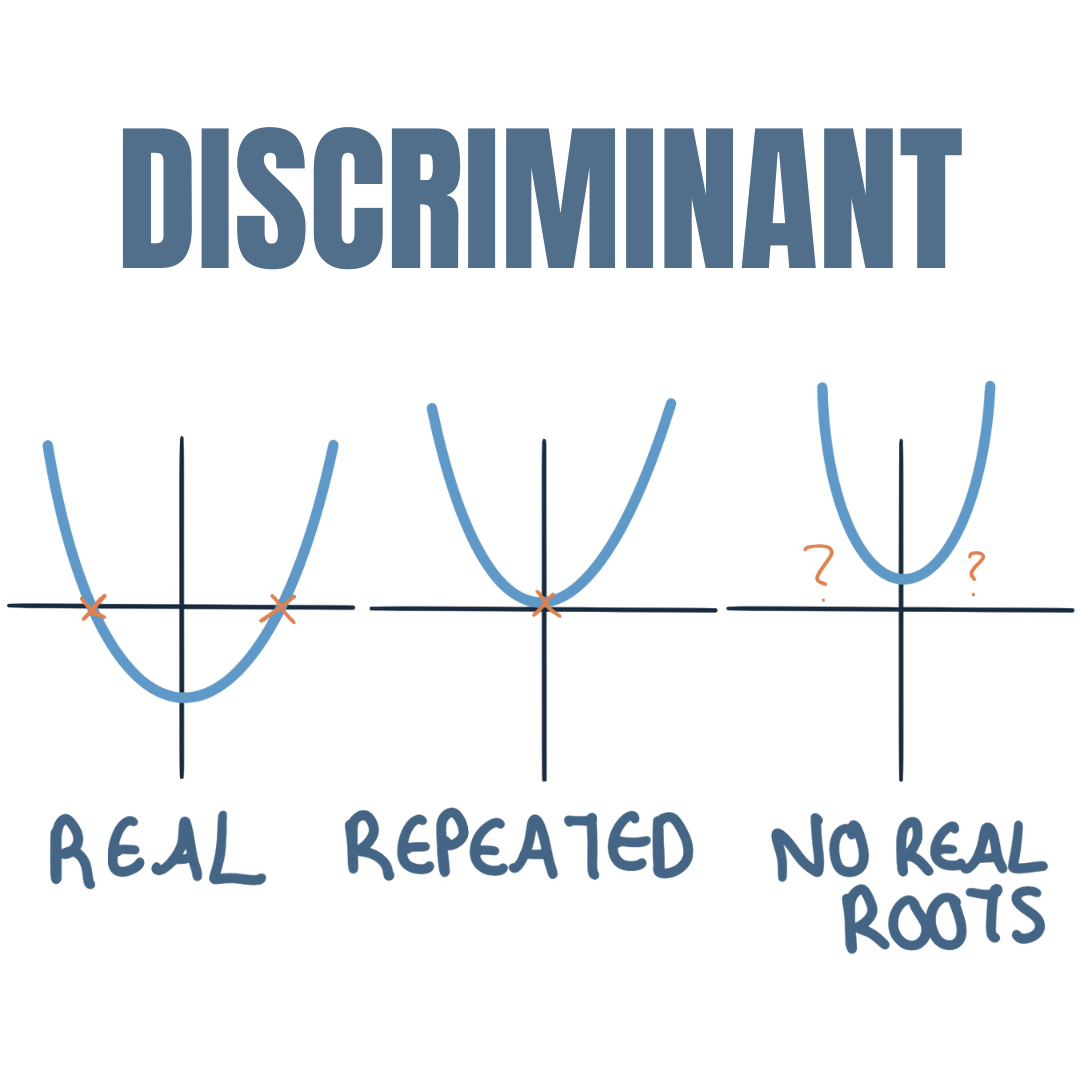
There are 3 types of roots:

THE DISCRIMINANT
It is possible to determine what roots the function will have without drawing a graph or solving the equation by using the discriminant.
Discriminant = ∆ (we use this symbol to represent it)
Referring to the quadratic equation, the discriminant is the part under the square root.

FINDING THE TYPES OF ROOTS USING THE DISCRIMINANT
If…
- ∆ > 0 – 2 distinct real numbers
- ∆ < 0 – No real roots
- ∆ = 0 – Repeated roots / equal roots
WHY?
In order to understand why this is true, it is useful to substitute these values into the quadratic equation and see what happens.

The ± is still there which means you will get 2 answers.

The ± disappears so there will only be 1 answer.

The square root is negative which means the answer won’t be real numbers.
Have any questions? Leave a comment below!

Leave a Reply